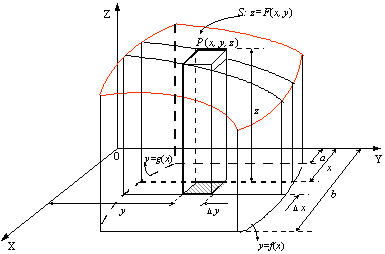
The Volume as a Double Integral
Problem 1
Find the general formula as a double integral in rectangular
coordinates that allows to find the volume between the lines x
= a, x = b, the curves
y = g(x), y = f(x),
and the surfaces z = 0, z = F(x,
y).
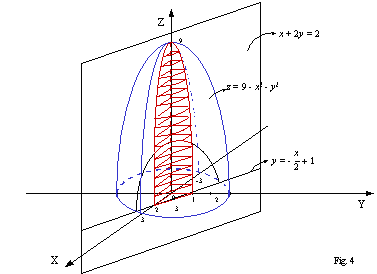
Problem 2
Using a double integral in rectangular coordinates, determine
the volume of the solid in the first octant under the surface z
=  and beside the plane x + 2y = 2.
and beside the plane x + 2y = 2.